Summary
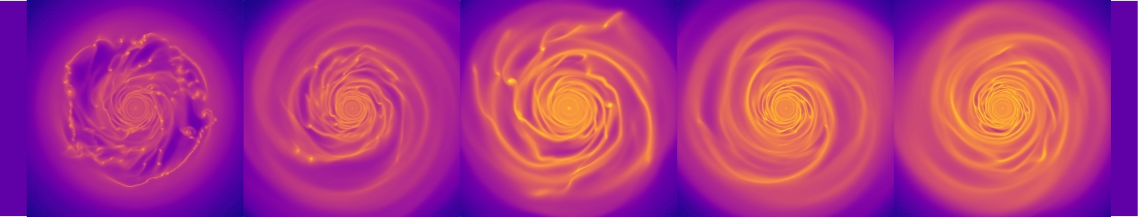
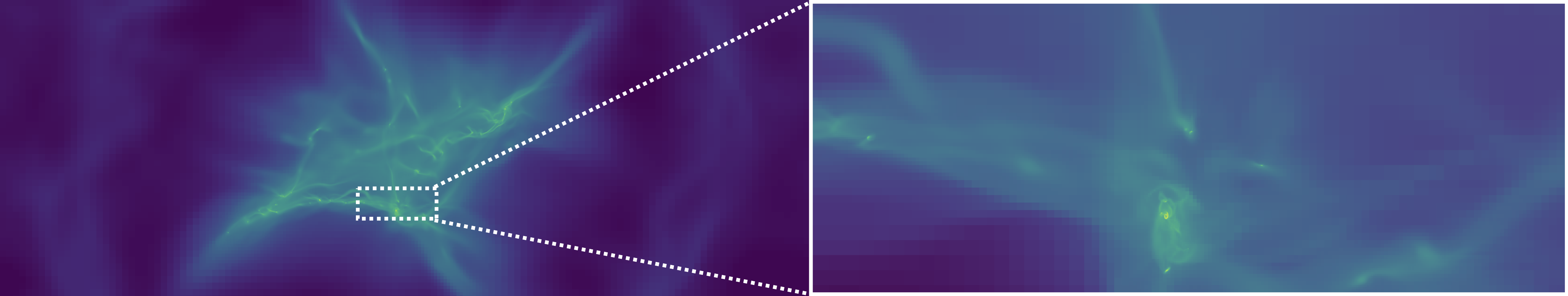
The Extreme Horizon simulation (EH) is performed with the adaptive mesh refinement code RAMSES (Teyssier 2002) using the physical models from Horizon-AGN (Dubois et al. 2014). The spatial resolution in the CGM and IGM is largely increased compared to Horizon-AGN, while the resolution inside galaxies is identical, at the expense of a smaller box size of $50 \; \textrm{Mpc}\cdot\textrm{h}^{-1}$. The control simulation of the same box with a resolution similar to Horizon-AGN is called Standard-Horizon (SH). EH and SH share initial conditions realized with mpgrafic (Prunet et al. 2008). These use a $\Lambda\textrm{CDM}$ cosmology with matter density $\Omega_{\textrm{m}} = 0.272$, dark energy density $\Omega_{\Lambda} = 0.728$, matter power spectrum amplitude $\sigma_{8} = 0.81$, baryon density $\Omega_{\textrm{b}} = 0.0455$, Hubble constant $\textrm{H}_{0} = 70.4 \; \textrm{km}\cdot\textrm{s}^{-1}\cdot\textrm{Mpc}^{-1}$, and scalar spectral index $\textrm{n}_{\textrm{s}} = 0.967$, based on the WMAP-7 cosmology (Komatsu et al. 2011). The Extreme Horizon simulation was performed on 25 000 cores of the AMD-Rome partition of the Joliot Curie supercomputer at TGCC and it partly used the Hercule parallel I/O library (Bressand et al. 2012; Strafella & Chapon 2020). It is being run down to $z\simeq 1.0$.Resolution strategy
The Standard-Horizon simulation uses a $512^{3}$ coarse grid, with a minimal resolution of $100 \; \textrm{kpc}\cdot\textrm{h}^{-1}$ as in Horizon-AGN. Cells are refined up to a resolution of $\simeq 1\; \textrm{kpc}$ in a quasi-Lagrangian manner: any cell is refined if $\rho_{\textrm{DM}} \Delta\textrm{x}^{3} + (\Omega_{\textrm{b}}/\Omega_{\textrm{DM}})\rho_{\textrm{baryon}}\Delta x^{3} > \textrm{m}_{\textrm{refine},\textrm{SH}}\textrm{M}_{\textrm{DM},\textrm{res}}$, where $\rho_{\textrm{DM}}$ and $\rho_{\textrm{baryons}}$ are dark matter (DM) and baryon densities respectively in the cell, $\Delta x^{3}$ is the cell volume, and $\textrm{m}_{\textrm{refine},\textrm{SH}}=80$. The $\Omega_{\textrm{b}}/\Omega_{\textrm{DM}}$ factor ensures that baryons dominate the refinement condition as soon as there is a baryon overdensity. This resolution strategy matches that of Horizon-AGN :| Comoving grid resolution $[\textrm{kpc}\cdot\textrm{h}^{-1}]$ | 97.6 | 48.8 | 24.4 | 12.2 | 6.1 | 3.05 | 1.52 | 0.76 |
| Physical grid resolution [kpc] ($z=2$) | 47 | 23.5 | 11.7 | 5.8 | 2.9 | 1.5 | 0.7 | 0.3 |
| Volume fraction (EH) ($z=2$) | - | 45% | 43% | 10% | 1% | 0.04% | $z<2$ | $z<2$ |
| Volume fraction (SH) ($z=2$) | 80% | 17% | 2% | 0.17 % | 0.013% | $5\times10^{-4} %$ | $z<2$ | $z<2$ |
| Volume fraction (HAGN) ($z=2$) | 77% | 19% | 2% | 0.2 % | 0.01% | $6\times10^{-4} %$ | $z<2$ | $z<2$ |